How To Play Baccarat Dragon
The easiest form of cover play is to simply bet on the banker and player bets back and forth with a small denomination of money. Then when the Dragon 7 becomes profitable, you play it with a large bet size in addition to the banker or player bet. This is a natural way to play, and it'll be much more difficult to spot what you're doing like this. The Dragon Bonus (DB) wager is arguably the most common and popular side bet for baccarat on the market. DB allows the gambler to not only wager that either the Banker side or Player side will win, but that the winning side will crush the losing side by a significant number of points.
- How do you play Dragon in Baccarat? Just like any bet in baccarat; you need to place the Dragon bet before the game begins. How often does a dragon hit in Baccarat? It has been suggested that the dragon is likely to come up about seven times (somewhat ironically) in an eight-deck shoe. What are the odds in EZ Baccarat? You face a house edge of.
- Dragon 7 Baccarat Side Bet Explained. Unlike Blackjack or Poker, Baccarat is a table game that doesn’t come in many variations. Yet, there are still Baccarat variants that provide players with fresh gaming experience. One of them is EZ Baccarat, the game variant we have already introduced on our gaming portal dedicated to this exciting card.
- Appendices
- Baccarat Analysis
- Miscellaneous
On This Page
Introduction
On This Page
How To Play Baccarat Dragon Odds
However, when Eliot Jacobson mentioned he had found the Dragon Bet in EZ Baccarat easily countable I was eager to cover it. As far as I know this topic has never been covered before. So I was quite happy when Eliot agreed to share the results of his analysis with my readers. Enjoy! — Wizard
Card Counting the Dragon Side Bet in EZ Baccarat
By Eliot Jacobson Ph.D., © 2011The Dragon Side Bet for EZ Baccarat is simple to describe. This side bet pays 40-to-1 if the dealer’s three-card total of 7 beats the player, otherwise the bet loses. Analysis of the wager consists of a straight forward cycle through all possible hands. Table 1 gives the analysis for eight decks, with the house edge of 7.611% appearing in the lower right cell.
Table 1
EZ Baccarat Dragon Side Bet
| Event | Pays | Combinations | Probability | Return |
|---|---|---|---|---|
| Winning Dragon | 40 | 112,633,011,329,024 | 0.022530 | 0.901350 |
| Losing Dragon | -1 | 4,885,765,264,174,330 | 0.977470 | -0.977470 |
| Total | 4,998,398,275,503,360 | 1.000000 | -0.076110 |
I first considered if the Dragon Side Bet was susceptible to a card counting methodology several months back. Intuitively, it seemed as though the wager was more probable to hit if there was an excess of 7 and 10 valued cards in the deck. In this case, the dealer would be more likely to draw 10-10 and hit to a 10-10-7 = 7. Later, as I read several Internet discussion boards, it became clear that others thought as I did. The conclusions were that if there was any vulnerability at all, it would come when 7’s and 10’s were in excess in the remainder of the shoe. It turns out this is not the case. The Dragon Side Bet is vulnerable to a card counting methodology, but the answer is surprising.
The key is that in order for the player to win the Dragon bet, the dealer has to draw a third card. This requirement trumps everything else. The cards that keep the dealer from drawing that third card most often are the 8 and the 9. As these cards are removed from the shoe, the edge moves quickly towards the counter’s favor. An excess of smaller cards is also helpful. The cards 1-7 are each cards that can move the dealer’s final total to 7 if he draws. Determining which of these low cards result in a final total of 7 most often is the key.
The methodology used in this study is familiar. The overall house edge for the game dealt from eight decks is 7.611%. By removing each card in turn from an eight-deck shoe, its effect on the house edge can be determined. This allows card counting systems to be developed. After arriving at candidate systems, computer simulations are run to see if these systems can generate an edge in practice. If there is an edge, the question then becomes if this is significant enough to become an opportunity for the advantage player.
Table 2 shows the number of winning and losing hands that result from removing one card from an eight-deck shoe, along with the house edge after removing that card.
Table 2
House Edge by Card Removed
| Card Removed | Winning Dragon | Losing Dragon | Total | House Adv. |
|---|---|---|---|---|
| 1 | 111,068,343,867,648 | 4,815,237,648,815,950 | 4,926,305,992,683,600 | -0.075620 |
| 2 | 110,900,807,733,248 | 4,815,405,184,950,350 | 4,926,305,992,683,600 | -0.077010 |
| 3 | 110,879,201,710,336 | 4,815,426,790,973,260 | 4,926,305,992,683,600 | -0.077190 |
| 4 | 110,686,449,371,648 | 4,815,619,543,311,950 | 4,926,305,992,683,600 | -0.078790 |
| 5 | 110,691,915,602,560 | 4,815,614,077,081,040 | 4,926,305,992,683,600 | -0.078750 |
| 6 | 110,618,934,007,296 | 4,815,687,058,676,300 | 4,926,305,992,683,600 | -0.079360 |
| 7 | 110,577,900,912,896 | 4,815,728,091,770,700 | 4,926,305,992,683,600 | -0.079700 |
| 8 | 111,654,703,169,536 | 4,814,651,289,514,060 | 4,926,305,992,683,600 | -0.070740 |
| 9 | 111,583,436,417,536 | 4,814,722,556,266,060 | 4,926,305,992,683,600 | -0.071330 |
| 10 | 111,112,191,215,104 | 4,815,193,801,468,490 | 4,926,305,992,683,600 | -0.075250 |
Table 2 allows us to compute the effect on the house edge for the Dragon bet by removing each card. Table 3 gives these results. The middle column (EOR) shows the change in house edge when the indicated card is removed. The final column (EOR x 1000) indicates potential card-counting tags to use in an optimal system.

Table 3
Effect of Removal
| Card Removed | EOR | EOR x 1000 |
|---|---|---|
| 1 | 0.000500 | 0.5 |
| 2 | -0.000900 | -0.9 |
| 3 | -0.001080 | -1.1 |
| 4 | -0.002680 | -2.7 |
| 5 | -0.002630 | -2.6 |
| 6 | -0.003240 | -3.2 |
| 7 | -0.003580 | -3.6 |
| 8 | 0.005380 | 5.4 |
| 9 | 0.004790 | 4.8 |
| 10 | 0.000860 | 0.9 |
Table 3 indicates the extreme importance of ridding the shoe of 8’s and 9’s. Also, the 7 is the most important card, as expected, to remain in the shoe. The other cards diminish in value as their pips go down, presumably because they are used in fewer and fewer situations to draw to a dealer total of 7. Working against intuition, the counter’s situation improves as zero-valued cards are removed from the deck.
Looking at the values in the last column of Table 3, and adjusting slightly to make it balanced, we get card counting “system 1” with tags (0.5, -0.9, -1.1, -2.7, -2.7, -3.3, -3.6, 5.4, 4.8, 0.9). The reader will most likely consider it daunting to use system 1 in practice. However, as a baseline counting system, it is worthwhile to see how it performs. In an effort to simplify this unwieldy system as much as possible, I also considered the card counting system with tags (0, 0, 0, -1, -1, -1, -1, 2, 2, 0). I’ll refer to this as “system 2.” This latter system is easily implemented by a counter of average skill level.
To gauge the effectiveness of each, I wrote a computer program to simulate using these two systems in live play. The game I simulated has the following shuffling and cut card rules:
- The game is dealt from a shoe with 8 decks.
- At the start of each shoe, a card is burned. Based on the value of the burn card, an additional number of cards are burned, equal to the value of the card.
- The cut card is placed 14 cards from the end of the shoe.
- After the cut card is dealt, one more round is dealt before shuffling.
Table 4 gives the results of a simulation of two hundred million (200,000,000) shoes.
Table 4
Simulation Results: 200M Shoes
| Item | System 1 | System 2 |
|---|---|---|
| Target Count | 10 | 4 |
| Expected Value | 7.315% | 8.032% |
| Standard Deviation | 6.456 | 6.567 |
| Frequency of Bet | 10.698% | 9.163% |
| Units Won per Shoe | 0.6361 | 0.5967 |
Update: 10/14/2011. Shortly after this article was published, I realized that I had made an error that caused me to significantly underestimate the player advantage. This error was caused by using single-deck estimations for the remaining cards in the shoe, rather than determining the exact true count based on the fractional decks remaining. I revised my simulation program and confirmed my updated results with Steve How of discountgambling.net. I apologize for any inconvenience I may have caused the reader.
It is clear from the last row of Table 4 that system 2, with tags (0, 0, 0, -1, -1, -1, -1, 2, 2, 0), performs remarkably well in comparison to its optimal cousin.
The person who uses system 2 should make the Dragon bet whenever the true count is +4 or higher. If he does so, then on average he will have an 8.03% edge over the house each time he makes the bet. This counter will have the opportunity to make the Dragon bet at or above the target true count on 9.16% of his hands. Given that the average shoe yields about 80 hands, the counter should be able to make, on average, about seven Dragon bets per shoe with the edge.
In dollar terms, if the house allows a Dragon bet up to $100 (say), then on a per-shoe basis the counter will average about $59.67 profit. The counter will earn about $8.03 per $100 wagered on the Dragon bet.
It is worthwhile to check that the simulated results for system 2 make sense combinatorially. One way to get a +4 true count off the top is to remove eight 8’s and eight 9’s from the deck. This will leave 400 cards remaining in the eight-deck shoe, with a running count of +32, for a true count of 4.16. In this case, combinatorial analysis gives a player edge of 1.0227%. Using a single deck, one way to get a +4 true count is to remove one 8 and one 9 from the deck. This leaves 50 cards with a +4 running count, giving a true count of 4.16. In this case, combinatorial analysis gives a player edge of 1.3114%. Because the player is making the Dragon bet at a true count of +4 and above, not just at +4, these computations represent a secondary confirmation of the simulated results.
How To Play Baccarat Dragon Ball Z
Cut card placement varies by casino, so it is worthwhile to investigate how the edge changes with the placement of the cut card. Table 5 gives statistics for all cut card placements from 14 cards to 52 cards, and then by half-deck increments up to four decks. A cut card placement at one deck, instead of at 14 cards, decreases the potential profit to the player by about 50%.
Table 5
Card Counting Statistics by Cut Card DepthExpand
| Cut Card Depth | Trigger Count | Hands per Shoe | Expected Value | Standard Deviation | Bet Frequency | Percent of Shoes Played | Profit per Shoe (units) | Profit per Hour (60 hands) |
|---|---|---|---|---|---|---|---|---|
| 12 | 4 | 81.69 | 8.30% | 6.575 | 9.31% | 67.31% | 0.631 | 0.464 |
| 13 | 4 | 81.48 | 8.15% | 6.570 | 9.24% | 66.54% | 0.613 | 0.451 |
| 14 | 4 | 81.28 | 8.03% | 6.567 | 9.16% | 65.81% | 0.597 | 0.440 |
| 15 | 4 | 81.08 | 7.87% | 6.562 | 9.09% | 65.12% | 0.580 | 0.429 |
| 16 | 4 | 80.88 | 7.81% | 6.560 | 9.02% | 64.47% | 0.569 | 0.422 |
| 17 | 4 | 80.67 | 7.67% | 6.556 | 8.95% | 63.81% | 0.554 | 0.412 |
| 18 | 4 | 80.47 | 7.64% | 6.555 | 8.87% | 63.14% | 0.545 | 0.407 |
| 19 | 4 | 80.27 | 7.48% | 6.551 | 8.80% | 62.48% | 0.528 | 0.395 |
| 20 | 4 | 80.07 | 7.42% | 6.549 | 8.73% | 61.80% | 0.518 | 0.388 |
| 21 | 4 | 79.86 | 7.37% | 6.547 | 8.66% | 61.14% | 0.510 | 0.383 |
| 22 | 4 | 79.66 | 7.28% | 6.545 | 8.58% | 60.51% | 0.498 | 0.375 |
| 23 | 4 | 79.46 | 7.20% | 6.542 | 8.52% | 59.93% | 0.487 | 0.368 |
| 24 | 4 | 79.26 | 7.04% | 6.538 | 8.45% | 59.35% | 0.472 | 0.357 |
| 25 | 4 | 79.05 | 7.03% | 6.537 | 8.38% | 58.77% | 0.466 | 0.353 |
| 26 | 4 | 78.85 | 6.92% | 6.534 | 8.32% | 58.20% | 0.454 | 0.345 |
| 27 | 4 | 78.65 | 6.88% | 6.533 | 8.25% | 57.65% | 0.446 | 0.340 |
| 28 | 4 | 78.45 | 6.84% | 6.532 | 8.18% | 57.13% | 0.439 | 0.336 |
| 29 | 4 | 78.24 | 6.75% | 6.529 | 8.12% | 56.64% | 0.429 | 0.329 |
| 30 | 4 | 78.04 | 6.69% | 6.527 | 8.05% | 56.12% | 0.421 | 0.323 |
| 31 | 4 | 77.84 | 6.61% | 6.525 | 7.99% | 55.61% | 0.411 | 0.317 |
| 32 | 4 | 77.64 | 6.58% | 6.524 | 7.92% | 55.06% | 0.405 | 0.313 |
| 33 | 4 | 77.43 | 6.49% | 6.521 | 7.86% | 54.53% | 0.395 | 0.306 |
| 34 | 4 | 77.23 | 6.47% | 6.521 | 7.80% | 53.99% | 0.389 | 0.302 |
| 35 | 4 | 77.03 | 6.38% | 6.518 | 7.73% | 53.49% | 0.380 | 0.296 |
| 36 | 4 | 76.83 | 6.33% | 6.517 | 7.67% | 53.00% | 0.373 | 0.291 |
| 37 | 4 | 76.62 | 6.22% | 6.513 | 7.61% | 52.53% | 0.363 | 0.284 |
| 38 | 4 | 76.42 | 6.21% | 6.513 | 7.55% | 52.06% | 0.358 | 0.281 |
| 39 | 4 | 76.22 | 6.18% | 6.512 | 7.49% | 51.59% | 0.353 | 0.278 |
| 40 | 4 | 76.02 | 6.15% | 6.511 | 7.43% | 51.13% | 0.347 | 0.274 |
| 41 | 4 | 75.81 | 6.10% | 6.510 | 7.37% | 50.70% | 0.340 | 0.269 |
| 42 | 4 | 75.61 | 5.97% | 6.506 | 7.31% | 50.29% | 0.330 | 0.262 |
| 43 | 4 | 75.41 | 6.05% | 6.508 | 7.25% | 49.85% | 0.330 | 0.263 |
| 44 | 4 | 75.21 | 5.97% | 6.506 | 7.19% | 49.40% | 0.323 | 0.257 |
| 45 | 4 | 75.00 | 5.92% | 6.504 | 7.13% | 48.95% | 0.317 | 0.253 |
| 46 | 4 | 74.80 | 5.81% | 6.501 | 7.07% | 48.48% | 0.307 | 0.246 |
| 47 | 4 | 74.60 | 5.80% | 6.501 | 7.01% | 48.03% | 0.304 | 0.244 |
| 48 | 4 | 74.40 | 5.72% | 6.498 | 6.95% | 47.60% | 0.296 | 0.239 |
| 49 | 4 | 74.19 | 5.68% | 6.497 | 6.90% | 47.19% | 0.291 | 0.235 |
| 50 | 4 | 73.99 | 5.68% | 6.497 | 6.84% | 46.77% | 0.287 | 0.233 |
| 51 | 4 | 73.79 | 5.63% | 6.496 | 6.78% | 46.36% | 0.282 | 0.229 |
| 52 | 4 | 73.59 | 5.62% | 6.495 | 6.73% | 45.95% | 0.278 | 0.227 |
| 1.5 decks | 4 | 68.32 | 4.79% | 6.470 | 5.37% | 36.51% | 0.176 | 0.154 |
| 2 decks | 4 | 63.06 | 4.16% | 6.451 | 4.20% | 28.71% | 0.110 | 0.105 |
| 2.5 decks | 4 | 57.79 | 3.64% | 6.436 | 3.19% | 22.09% | 0.067 | 0.070 |
| 3 decks | 4 | 52.53 | 3.22% | 6.423 | 2.34% | 16.44% | 0.039 | 0.045 |
| 3.5 decks | 4 | 47.27 | 3.13% | 6.420 | 1.62% | 11.70% | 0.024 | 0.030 |
| 4 decks | 4 | 42.00 | 2.74% | 6.409 | 1.05% | 7.79% | 0.012 | 0.017 |
This analysis shows that in theory the Dragon Side Bet in EZ Baccarat is an advantage play opportunity using a card counting methodology. In my opinion, however, given the high variance and low return, card counting is not a practical threat to the game.
About the Author
For more information on Eliot, or to contact him, visit http://ijmp.org/ .
Related Pages
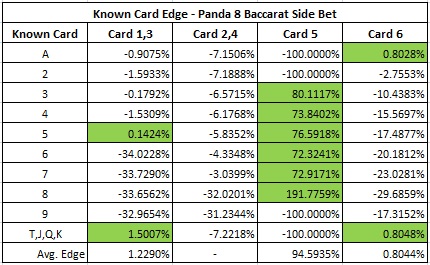
Please also see my own card counter vulnerability study of the Panda 8 side bet in EZ Baccarat.How Do You Play Baccarat
Written by:Michael Shackleford